DANotes: Stress etc: Load building blocks

Elementary load building blocks

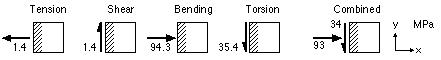
We refer to tension, shear, bending and torsion as elementary load building blocks because the loading which is applied to most components is a combination (superposition) of these four - for example, a rotating shaft which transmits power is subjected not only to torsion, but usually also to bending and to direct shear. The similarities and differences between the load building blocks are illustrated here.

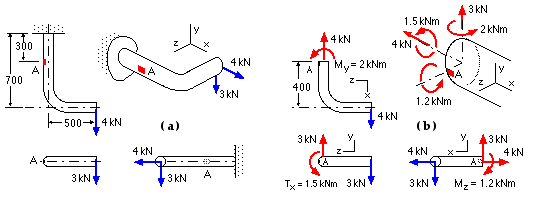
This first example applies the building blocks to the analysis of a bent cantilever.

EXAMPLE

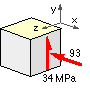
The bent cantilever, shown at ( a) below, is made from 60 mm diameter rod. Determine the stress components at the surface element A lying in the horizontal plane of the cantilever's centreline.

cantilever example
The stress resultants at the cross-section which contains A are readily established by the free bodies shown in ( b). Evaluating the stress components from the building block equations :-

Tension P = 4 kN
σ = P / A = 4E3 * 4 / π * 60² = 1.4 MPa Note, the practical unit of stress is MPa
Shear V = 3 kN
Since we know the correct distribution of shear stress (see any Mechanics of Materials text under bending shear) we shall use this in preference to the simplistic uniform model. As A lies in the neutral plane it will be subjected to the maximum shear stress, which for a circular cross-section is :-
τ = 4V / 3A = 4 * 3E3 * 4 / 3 π * 60² = 1.4 MPa Don't forget to monitor units
Bending M_z = 1.2 kNm
Since A lies on the neutral plane there is no stress at A attributable to this moment component.
Bending M_y = 2 kNm
σ = M z / I = 2E6 * 30 * 64 /π * 60⁴ = 94.3 MPa compressive at A by inspection.
Torsion T_x = 1.5 kNm
τ = T r / J = 1.5E6 * 30 *32 /π * 60⁴ = 35.4 MPa downward as sketched in ( b) by inspection.

Alternatively, the components of the equilibrating moment at A might have been found by vector algebra:

M = - r x F = - [ 0.4 0 -0.5 ]' x [ 4 -3 0 ]' kN.m = [ 1.5 2 1.2 ]' kNm = [ M_x M_y M_z ]'

where M_x here is in fact the torque T_x identified above. Care should be exercised in using such a vector approach not to lose sight of the physical significance of the numbers and senses.

The stress components from the building blocks are sketched and then combined by inspection below.

stress superposition

The above example illustrates an important general conclusion :

- if bending is present then direct normal stresses are usually insignificant,

- if torsion is present then direct shear stresses are usually insignificant.

Like all general conclusions this has to be applied with some discretion. It will not apply near a beam's simple support for example - however this region of the beam is unlikely to be critical.

The load building blocks which occur in a particular component are not always immediately obvious. The next example, in the realm of design, illustrates how to go about discovering blocks' occurrence. It also introduces another load building block - crushing aka bearing due to direct contact between two bodies, and emphasizes the need to identify ALL potential failure modes.
After all, the designer must define all dimensions . . . . and materials, and tolerances, and finishes, etc. . . . . the designer is responsible for everything.

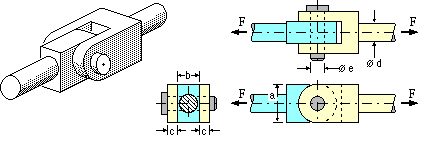
This next example applies the building blocks to the design (synthesis) of a cotter joint.

EXAMPLE

Select suitable dimensions for the separable cotter joint illustrated, which transmits a tensile load, F. The allowable stresses, S_t in tension and S_s in shear, are known for each ductile component.

cotter joint
The first step is to evaluate the external effects on each component - then, for each component, the force paths can be traced, the possible modes of failure identified and the corresponding design equations applied. To illustrate the technique, we shall consider only the left component; the right component is very similar. The joint is statically determinate, so the free body of the component with simplified lines of force will be as shown in Figure A below.

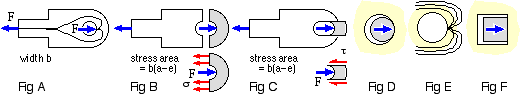
joint failure modes
Some of the failure modes are :-

Tension in rod end area = π d² /4 σ = 4 F/πd² ≤ S_t d ≥ √( 4F/πS_t)
Tension in way of hole, Fig B area = b ( a - e) σ = F/b(a-e) ≤ S_t b(a-e) ≥ F/S_t
Note that this is the most critical tensile location in the LH component's body
Shear of end ligament, Fig C area = b(a-e) approx τ = F/b(a-e) ≤ S_s b(a-e) ≥ F/S_s

In practice, we expect interaction between tension and shear (and contact phenomena, see below) around the end of the component, which, together with stress concentration, would lead to a more complex stress state, failure mechanism and fracture surface. However the simple approach used here is acceptable, provided suitably large factors of safety (ie. of ignorance) are incorporated in the allowable stresses used in design. Such an elementary approach usually leads to wasteful overdesign of components, and it is to avoid this that we try to employ more realistic mathematical models.

Bearing in hole, Fig D.

If the two contacting members are rigid then the contact area is zero and the stresses infinite. In reality both contacting components deform slightly resulting in a small contact area; actual stresses are therefore finite but large as evidenced by the bunched force paths in Fig E. We shall examine contact stresses (another load building block) in more detail later; for the time being we use the simplified concept of a critical crushing or bearing pressure, p_c, which is taken to be uniform over the equivalent projected area Fig F. This critical pressure is obviously very much less than the material strengths, however the simple approach is reasonable provided we know from separate experiments the critical pressure which the weaker of the two materials is capable of sustaining. We then have :-
Area = eb projected p = F/eb ≤ p_c known e b ≥ F/p_c

. . . . and so on. When all failure modes have been investigated (and these would include buckling, excessive deflection, crack growth rate etc, if relevant, not just strength as above) then a set of dimensions which satisfies all the inequalities would be chosen as a design basis. Some dimensions may have to be increased for other practical reasons - a part might be too flimsy for clamping during manufacture, or too prone to damage during transportation, unless dimensions were greater than the above safe minima.

In this latter example we assumed that the failure mechanisms were separate - tension, shear etc. occur at disparate points. resultant stress

The more general state of affairs is typified by the first example in which a number of load building blocks occur simultaneously at the element of interest. That example demonstrated how the stresses from the various load building blocks are combined to define the stress state at the element.
So far, so good - but if we want to determine the degreee of safety at this element (given the material strength) we face problems. It was easy to weigh up the safety of the tensile bar for example (equation 1b) because both the bar and the specimen which provided the material strength (S_y or S_u) were loaded purely in tension. But that's not the case here - while the tensile specimen is loaded in pure tension, the component certainly is not. Shear loading also is present.
There is no theoretical rationale which we can adopt to correlate these 'different' loadings and assess safety - instead, we have to employ a suitable empirical failure theory.

Suppose we were analysing a body on which loading was not known completely; we might ascertain that the stress components in the x- and y- directions were zero. Would we be justified in deducing from this that there was no load on the body and 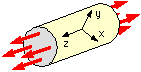tensile specimen therefore that the safety factor was infinite?
Of course not! as a quick appraisal of a tensile bar loaded in the z-direction will confirm. Degree of safety must be intrinsic to the loaded body and completely independent of whichever Cartesian axes we happen to choose for the analysis.
So before we can look at failure theories we must be able to assess the stress components as the Cartesian system is rotated, and to evaluate therefrom the stresses which are intrinsically relevant to safety issues. It's time to review the concepts of this stress resolution.

Tension P = 4 kN
	σ = P / A = 4E3 * 4 / π * 60² = 1.4 MPa Note, the practical unit of stress is MPa
Shear V = 3 kN
	Since we know the correct distribution of shear stress (see any Mechanics of Materials text under bending shear) we shall use this in preference to the simplistic uniform model. As A lies in the neutral plane it will be subjected to the maximum shear stress, which for a circular cross-section is :- τ = 4V / 3A = 4 * 3E3 * 4 / 3 π * 60² = 1.4 MPa Don't forget to monitor units
Bending M_z = 1.2 kNm
	Since A lies on the neutral plane there is no stress at A attributable to this moment component.
Bending M_y = 2 kNm
	σ = M z / I = 2E6 * 30 * 64 /π * 60⁴ = 94.3 MPa compressive at A by inspection.
Torsion T_x = 1.5 kNm
	τ = T r / J = 1.5E6 * 30 32 /π 60⁴ = 35.4 MPa downward as sketched in ( b) by inspection.

Tension in rod end	area = π d² /4	σ = 4 F/πd² ≤ S_t	d ≥ √( 4F/πS_t)
Tension in way of hole, Fig B	area = b ( a - e)	σ = F/b(a-e) ≤ S_t	b(a-e) ≥ F/S_t
Note that this is the most critical tensile location in the LH component's body
Shear of end ligament, Fig C	area = b(a-e) approx	τ = F/b(a-e) ≤ S_s	b(a-e) ≥ F/S_s