Stress concentration
We shall use a tightened nut and bolt, in which the detailed stress variation is extremely complicated, to illustrate some of the simplifying assumptions which are usually made in routine analysis and design.
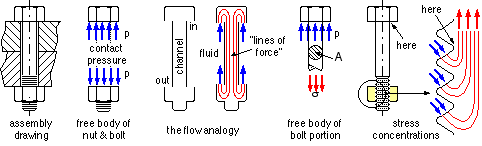
The leftmost sketch below shows an assembly comprising a nut and bolt which fasten two components together. The nut and bolt pair can be regarded as a sub-assembly or component in its own right. Before any identification of the stresses can be attempted, all external effects on this sub-assembly must be known. A free body of the nut and bolt demonstrates that the external load on them is the force P due to contact over annular areas with the two fastened components.
The flow analogy is useful when visualising how stress is transmitted through a loaded component. In the analogy, lines of force (or force paths ) in the component are likened to streamlines in a fluid channel whose shape is similar to that of the component - fluid enters and leaves the channel at locations which correspond to the areas where the external loads are applied to the component.
- A complicated streamline pattern infers a complex stress situation.
- Stresses are low where the streamlines are widely spaced.
- Stresses are high where the streamlines are bunched together due to geometric shape variations - the more sudden these variations, the higher the local stresses. This last is known as stress concentration. Geometric irregularities give rise to non-uniform stresses.
A free body of portion of the bolt which includes the bolt head shows that the external load P is equilibrated by stress σ in the shank. If the free body boundary cuts the shank in the middle then the stress is uniform across the shank cross-section of area A - that is σ = P/A.
But if the boundary lies close to the head or to the thread and nut, then stress concentration occurs and the maximum stress is greater than P/A.
If it is required to calculate the elastic extension, δ, of a bolt whose length L is relativey large, then a first approximation neglects stress concentration to obtain δ = Lε = L(σ/E) = LP/AE = P/k where the stiffness , k, of the bolt is defined as k = AE/L.

If the accent is on bolt safety rather than on deflection, then the core area Ac should be used for stress calculations - this minimum area in the force transmission path is formed by a transverse plane cutting the bolt thread between the plain shank and the nut's contact face.
The rightmost sketch above employs similar reasoning to illustrate details of the complex transfer of force across the thread into the bolt, together with the high stress concentration in way of the thread root, which may lead to localised yielding.
Static indeterminacy
The external effects on each member of an assembly - the loads and deformations - must be resolved before stresses and strains internal to the member can be examined. If the assembly is statically indeterminate then resolution is carried out by a three-pronged attack :-
- Equilibrium of all the members must be assured, so free bodies of the individual members are drawn, complete with known and unknown loads consistent with the physical contact between the various members. The equilibrium equations are written down.
- Compatibility considers all the members' deformations, and the constraints between these deformations which are necessary to preserve the geometric integrity of the assemblage.
This step is carried out by sketching the assembly in the free and in the loaded conditions with member deformations exaggerated for clarity; the compatibility equations which state the integrity constraints are then written down by inspection.
A very common error is not to pay sufficient attention to compatibility.
- Definition of the constitutive law for each of the members which undergoes significant deformation enables resolution of the indeterminacy. The constitutive law for each member inter-relates the member's external load and deformation. If the member is elastic, the constitutive law is based upon :-
( 2) εx = [ σx - ν ( σy + σz ) ] / E + αt ; γxy = τxy / G etc.
- which includes a thermal expansion term due to the temperature rise t.
If a component is loaded uniaxially and uniformly then, like the bolt above, equations (2) degenerate to :-
( 2a) δ = P / k + L α t
- where δ is the extension of the component whose length is L and cross-sectional area is A, and on which the tensile load is P; the axial stiffness of the component is k = AE/L.
If the load is compressive or if the deformation is a shortening then the signs of the various terms of ( 2a) will have to be changed by inspection or by the positive tensile convention.
If a component is bent then its stiffness (load/deflection) follows from beam theory.
The technique is applied to an assemblage of uniaxially loaded components in this example.
Two- or three-component assemblies are examined here, but statically indeterminate assemblies with many components are by no means uncommon. The Appendix considers one class of such assemblages in which two loaded members are joined by a number of identical connectors in parallel, the problem being to ascertain the proportion of the total load transferred through each connector. Examples of this class include :
- a chain drive in which the chain tension is transferred into the sprocket wheel through a number of identical sprocket teeth, and
- a threaded joint where the bolt load is transferred into the nut by a number of thread turns.
This latter case may be appreciated qualitatively by imagining a rubber bolt screwed into a steel nut. When the bolt is pulled it stretches noticeably, but the relatively rigid nut prevents the bolt stretching inside the nut . . . and if the bolt suffers no strain then it suffers no stress - that is, no load is transferred through the bolt in way of the nut. It is concluded therefore that all the load is transferred in the first thread and the threads are not loaded equally. The implications of this on the safety of the threads is obvious.
Although most indeterminate assemblages do not contain rubber components, load distribution nevertheless depends markedly upon the relative stiffnesses of the various components.
That's why we generally much prefer statically determinate assemblages - the loads depend solely on statics; we know where we are with the loads and don't need large factors to account for our ignorance.
Having ascertained the external loads on a component either from statics or from resolution of an indeterminacy, it's now time to consider the load building blocks which are present and to ascertain the corresponding stresses . . . .
 Copyright 1999-2005 Douglas Wright,
doug@mech.uwa.edu.au
Copyright 1999-2005 Douglas Wright,
doug@mech.uwa.edu.au
last updated May 2005

![]()