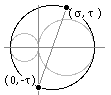Traditional analysis

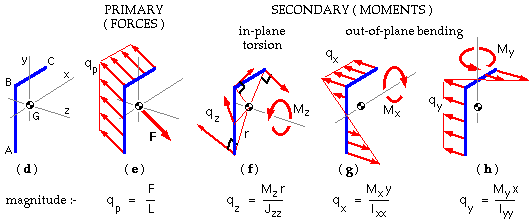
A typical planar joint run comprising two straight segments AB and BC is shown at ( d) below. A convenient Cartesian system is erected at the run's centroid with the run lying in the x-y weld plane. In the sketch, the external loads on the loaded member have been transferred to the run centroid to constitute the known load   F, M on the joint - it is this load which is equilibrated by the force intensity   q along the run.

The traditional approach treats force intensity along a weld run analogously to stresses in a beam, resolving   q into :

• The primary intensity   q_p is uniform along the run and equilibrates the centroidal force component of the load :   q_p = - F/L where   L is the total length of the run. Thus the sense of q_p is opposite to that of F as indicated in ( e).
  It is often more straightforward to resolve   F and   q_p into their components   q_px = F_x /L and so on.
  Primary intensity is analogous to the direct stress in a beam's cross-section which also is uniform - eg.   σ_d = F/A.
• The secondary intensity varies linearly along the run and equilibrates the moment load   M. The secondary intensity is split into two components which are each analogous to a basic load building block :
  • The in-plane or torsional component of secondary intensity,   q_z of Fig ( f), equilibrates the moment which is orthogonal to the weld plane,   M_z. This moment is analogous to the torque T in a shaft or beam, so M_z is often referred to as the "torque" on the joint.
    At any point on the run the torsional intensity, like torsional shear stress in a shaft cross-section :
    • lies in the weld plane,
    • is directed perpendicularly to the radius   r from the centroid to the point in question, and in a sense opposing M_z, and
    • is of magnitude given by   q_z = M_z r/J_zz, ie. the torque load building block with appropriate units (N/mm).
  • The out-of-plane or bending component of secondary intensity equilibrates the moment lying in the weld plane - in the general case this latter consists of two Cartesian components, eg. M_x and M_y in Figs ( g), ( h) above.
    At any point on the run the bending intensity - say q_x of ( g) - like normal bending stress in a beam cross-section :
    • is orthogonal to the weld plane,
    • is directed perpendicularly to the coordinate   y from the centroid to the point in question, and in a sense opposing M_x, and
    • is of magnitude given by   q_x = M_x y/I_xx, ie. the bending load building block with appropriate units (N/mm).

The total force intensity   q is the vector sum of primary and secondary components. Vector summation senses must be deduced by inspection, which can be rather tedious. The procedure is exemplified below.

• joint runs which are planar, made up of straight segments, and symmetric;
• loading which is simple, eg. consisting of a single force and moment component, because otherwise vector recomposition like that of the above example can become very tedious.

Throat stresses and joint safety

The following assumptions are made :-

• failure of joints in practice occurs most commonly in the throat so any safety analysis need consider only the throat
• in spite of the extreme stress concentration at the root geometric singularity, the stresses in the critical throat plane may be considered uniform.

The resultant intensity in global terms   q = [ q_x q_y q_z ]' has been evaluated as above for a certain weld element of length   δL. The intensity is resolved into components local to the element   q = [ q₁ q₂ q₃ ]' in Fig ( j) below - the 1-direction being longitudinal ie. along the run, and the 2- and 3-directions normal to the fillet faces.

For the point C of the preceding example, the 1-2-3 directions local to the joint at C happen to correspond to the global x-y-z senses, so :
      q_C   =   [ q₁   q₂   q₃ ]'   =  [ q_x   q_y   q_z ]'   =   [ 18.6   -19.4   -324.5 ]' N/mm
By inspection at the point A on the other hand :
      q_A   =   [ q₁   q₂   q₃ ]'   =   [ q_y   -q_x   q_z ]'   =   [ -7.6   -1.0   -27.0 ]' N/mm

The equilibrating components on the loaded member face of the element in ( j) demonstrate that   q₁ is essentially different to   q₂ and   q₃ as these latter appear as shear and normal effects on adjacent fillet faces whereas   q₁ manifests itself as shear on both faces. To correlate throat stresses with the known intensity of ( j), we consider equilibrium of a free body of the top half of the fillet, one of whose boundaries is the throat, Fig( k). The uniform stress over the throat consists of a component   σ normal to the throat plane, together with two mutually perpendicular shear components lying in the throat plane - the longitudinal shear component   τ_l and the transverse shear component   τ_t.

Since   δF =   q.δL =   σ.δA   and the throat width   a = w/√2,   force equilibrium of the free body ( k) requires :
( ii)       τ_l aδL   =   q₁ δL   ;       τ_t aδL   =   ( q₂ + q₃ ) δL/√2   ;       σ aδL   =   ( q₂ - q₃ ) δL/√2  

Applying the distortion energy failure theory to this plane stress state, the equivalent uniaxial stress in the throat of the fillet element,   σ_e, is given by :-
          σ_e²   =   σ² + 3 τ²   =   σ² + 3 ( τ_l² + τ_t² )       using Pythagoras

Substituting for the stresses from ( ii) and introducing the concept of the scalar equivalent force intensity q_e which causes the same failure tendency as the given  q yields :-

( 1)       w σ_e   =   2 q_e       where   q_e   =   √( ³/₂q₁² + q₂² + q₂ q₃ + q₃² ) ;       σ_e   =   S/n

                              in which   S is the strength of the weld material and   n is the safety factor at the fillet. Note that ( 1) contains the four indispensible ingredients of a design equation - safety (n), dimensions (the constant fillet size, w), material (characterised by strength S) and loading characterised by the intensity (q).

Like the beam, the final analysis step involves a search along the run to determine the most critically loaded fillet and hence the minimum safety factor of the joint as a whole.